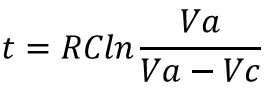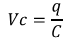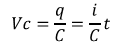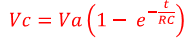1-Measure the voltage Vc across the capacitor vs. time

Fixing a value for the voltage Va (slider on power supply).

Choose a value for C (slider C).

Press RESET.
Press START/STOP button.

The capacitor begins to charge: the pointer of the voltmeter begins to rise.

Measure the time when the voltmeter reaches a predetermined value of Vc. If the pointer of the voltmeter rises very quickly, You can press the STOP/START button to read the time. To continue press START/START

Transfer the experimental data to an Excel spreadsheet and analyse the results. Click here to download and see an example (charging_capacitor.xlsx)

2-Measures the time that the capacitor takes to reach a voltage equal to 63% of Va.

Compare this time with the time constant t= RC.
Repeat measurements by selecting other values of Va and C (R is set at 1 MW)

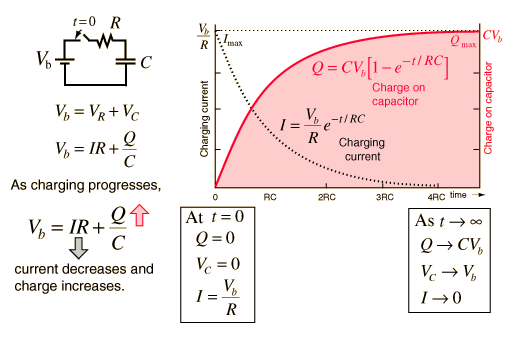
Charging a Capacitor

When a battery is connected to a series resistor and capacitor, the initial current is high as the battery transports charge from one plate of the capacitor to the other. The charging current asymptotically approaches zero as the capacitor becomes charged up to the battery voltage. Charging the capacitor stores energy in the electric field between the capacitor plates. The rate of charging is typically described in terms of a time constant RC.

https://en.wikipedia.org/wiki/Capacitor

 

(You can translate or you can write a new article suitable for your pupils)

Teoria cenni introduttivi

La tensione Vc ai capi di un condensatore dipende dalla carica q in essa immagazzinata e dalla sua capacità C secondo la formula:

Se carichiamo il condensatore con una corrente costante i, la carica immagazzinata, dopo un certo tempo t, sarà uguale a

e la ddp del condensatore sarà proporzionale al tempo trascorso.

Se il condensatore viene caricato con una tensione costante Va attraverso un resistore R la tensione ai capi del condensatore aumenterà al passare del tempo:

Il prodotto R*C che appare nella formula viene spesso indicato con la lettera greca t (tau) ed è chiamato “costante di tempo”. La tensione Vc aumenta con il tempo, ma non in modo proporzionale. All’inizio si carica velocemente poi man mano si carica sempre più lentamente.

 

t	Vc
0.1t	10% of Va
0.2t	18% of Va
0.5t	39% of Va
1.0t	63% of Va
2.0t	86% of Va
5.0t	99% of Va

Dalla tabella sopra si vede che dopo un tempo pari a 0,5t il condensatore si carica a circa il 39% della tensione massima (Va). Dopo un tempo pari a 1t la tensione raggiungerà un valore pari a circa il 63% di Va. Poichè il condensatore si carica sempre più lentamente, teoricamente non dovrebbe mai raggiungere il valore di Va. In pratica si considera il condensatore carico dopo trascorso un tempo pari a 4-5t

 

 

USEFUL FORMULAS

Charging a capacitor

If a capacitor is charged with a constant voltage Va through a resistance Rc, the voltage (Vc) reached by the capacitor after a time t is:

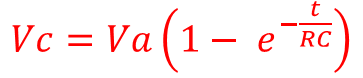
To reach a voltage Vc, the capacitorwill take a time equal to: